PSAT Math Multiple-Choice Question 700: Answer and Explanation

Question: 700

Triangle XYZ has a right angle for angle Y and has side lengths of 24 for XY and 26 for XZ . For a triangle that is similar to XYZ , what would be the value of the tangent of its smallest angle?

• A.
• B.
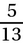
• C.
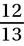
• D.

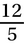
Correct Answer: A

Explanation:

(A) We can solve for the unknown side using the Pythagorean theorem:

a² + b² = c²

It follows that

Similar triangles have the same trigonometric ratios because the similar sides simplify to their lowest multiples.

The smallest angle in this triangle is angle X, as it is across from the shortest side length.

Therefore, a similar triangle will have a tangent of , which simplifies to , choice (A).

Alternatively, you could have saved yourself some time by noticing that this is just a multiple of a 5-12-13 Pythagorean triple. The two known sides are 2(12) = 24 and 2(13) = 26. The only side length we were missing was the 5 side, which has a measure of:

2(5) = 10.