PSAT Math Multiple-Choice Question 684: Answer and Explanation

Question: 684

What relationship must exist between the constants a, b, and c for the equation ax ² + bx + c = 0 to have only real solutions?

• A. b² ≤ 4ac
• B. b² ≥ 4ac
• C. 2a > -b
• D. Cannot be determined

Correct Answer: B

Explanation:

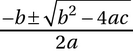(B) Recall that the quadratic formula tells us that solutions to the quadratic equation ax² + bx + c = 0 are x = Thus, for a quadratic function to only have real solutions, we need to be real numbers. This means that the expression under the square root, b² - 4ac, must be positive or 0. (If the value underneath the square root sign is negative, the solutions will be imaginary since the square roots of negative numbers are imaginary.)

In other words:

b² - 4ac ≥ 0

If we add 4ac to both sides:

b² ≥ 4ac

The answer is choice (B).